Page 65 - 4234

Σ ‖ base Σ  base
circle ellipse rectangle
Figure 7.4

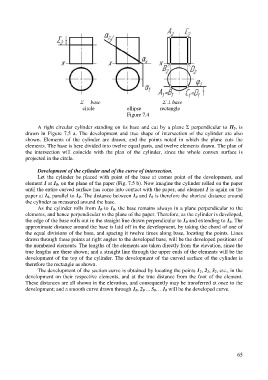
A right circular cylinder standing on its base and cut by a plane Σ perpendicular to Π 2, is
drawn in Figure 7.5 a. The development and true shape of intersection of the cylinder are also
shown. Elements of the cylinder are drawn, and the points noted in which the plane cuts the
elements. The base is here divided into twelve equal parts, and twelve elements drawn. The plan of
the intersection will coincide with the plan of the cylinder, since the whole convex surface is
projected in the circle.

Development of the cylinder and of the curve of intersection.
Let the cylinder be placed with point of the base at corner point of the development, and
element 1 at 1 0, on the plane of the paper (Fig. 7.5 b). Now imagine the cylinder rolled on the paper
until the entire curved surface has come into contact with the paper, and element 1 is again on the
paper at 1 0, parallel to 1 0. The distance between 1 0 and 1 0 is therefore the shortest distance around
the cylinder as measured around the base.
As the cylinder rolls from 1 0 to 1 0, the base remains always in a plane perpendicular to the
elements, and hence perpendicular to the plane of the paper. Therefore, as the cylinder is developed,
the edge of the base rolls out in the straight line drawn perpendicular to 1 0 and extending to 1 0. The
approximate distance around the base is laid off in the development, by taking the chord of one of
the equal divisions of the base, and spacing it twelve times along base, locating the points. Lines
drawn through these points at right angles to the developed base, will be the developed positions of
the numbered elements. The lengths of the elements are taken directly from the elevation, since the
true lengths are there shown; and a straight line through the upper ends of the elements will be the
development of the top of the cylinder. The development of the curved surface of the cylinder is
therefore the rectangle as shown.
The development of the section curve is obtained by locating the points 1 2, 2 2, 3 2, etc., in the
development on their respective elements, and at the true distance from the foot of the element.
These distances are all shown in the elevation, and consequently may be transferred at once to the
development; and a smooth curve drawn through 1 0, 2 0 ... 5 0… 1 0 will be the developed curve.

65