Page 63 - 4234

7 INTERSECTIONS OF SOLIDS AND SURFACES

Line method: A number of lines are drawn on the lateral surface of one of the solids and in
the region of the line of intersection. Points of intersection of these lines with the surface of the
other solid are then located. These points will lie on the required line of intersection. They are more
easily located from the view in which the lateral surface of the second solid appears edgewise (i.e.
as a line). The curve drawn through these points will be the line of intersection.

Cutting-plane method: The two solids are assumed to be cut by a series of cutting planes. The
cutting planes may be vertical (i.e. perpendicular to the horizontal plane Π 1), edgewise (i.e.
perpendicular to the frontal plane Π 2) or oblique. The cutting planes are so selected as to cut the
surface of one of the solids in straight lines and that of the other in straight lines or circles.

7.1 INTERSECTIONS OF POLYHEDRON AND PLANE

Plane is the simplest of surfaces. There are two methods for finding intersections of
polyhedron and plane: edge method and face method.
Edge method is based on intersection of line and plane (Fig. 7.1).
Face method is based on intersection of planes (Fig. 7.1).

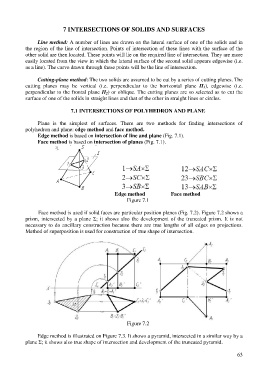
Edge method Face method
Figure 7.1

Face method is used if solid faces are particular position planes (Fig. 7.2). Figure 7.2 shows a
prism, intersected by a plane Σ; it shows also the development of the truncated prism. It is not
necessary to do ancillary construction because there are true lengths of all edges on projections.
Method of superposition is used for construction of true shape of intersection.

Figure 7.2

Edge method is illustrated on Figure 7.3. It shows a pyramid, intersected in a similar way by a
plane Σ; it shows also true shape of intersection and development of the truncated pyramid.

63