Page 64 - 4234

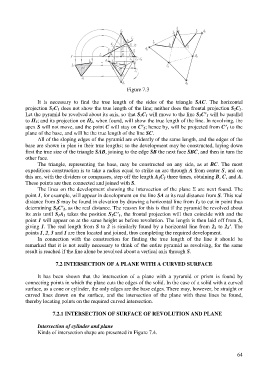
Figure 7.3

It is necessary to find the true length of the sides of the triangle SAC. The horizontal
projection S 1C 1 does not show the true length of the line; neither does the frontal projection S 2C 2.
Let the pyramid be revolved about its axis, so that S 1C 1 will move to the line S 1C’ 1 will be parallel
to Π 1; and its projection on Π 2, when found, will show the true length of the line. In revolving, the
apex S will not move, and the point C will stay on C’ 2; hence by, will be projected from C’ 1 to the
plane of the base, and will be the true length of the line SC.
All of the sloping edges of the pyramid are evidently of the same length, and the edges of the
base are shown in plan in their true lengths; so the development may be constructed, laying down
first the true size of the triangle SAB, joining to the edge SB the next face SBC, and then in turn the
other face.
The triangle, representing the base, may be constructed on any side, as at BC. The most
expeditious construction is to take a radius equal to strike an arc through A from center S, and on
this arc, with the dividers or compasses, step off the length A 1C 1 three times, obtaining B, C, and A.
These points are then connected and joined with S.
The lines on the development showing the intersection of the plane Σ are next found. The
point 1, for example, will appear in development on the line SA at its real distance from S. This real
distance from S may be found in elevation by drawing a horizontal line from 1 2 to cut in point thus
determining S 2C’ 2, as the real distance. The reason for this is that if the pyramid be revolved about
its axis until S 1A 1 takes the position S 1C’ 1, the frontal projection will then coincide with and the
point 1 will appear on at the same height as before revolution. The length is then laid off from S,
giving 1. The real length from S to 2 is similarly found by a horizontal line from 2 2 to 2 2’. The
points 1, 2, 3 and 1 are then located and joined, thus completing the required development.
In connection with the construction for finding the true length of the line it should be
remarked that it is not really necessary to think of the entire pyramid as revolving, for the same
result is reached if the line alone be revolved about a vertical axis through S.

7.2 INTERSECTION OF A PLANE WITH A CURVED SURFACE

It has been shown that the intersection of a plane with a pyramid or prism is found by
connecting points in which the plane cuts the edges of the solid. In the case of a solid with a curved
surface, as a cone or cylinder, the only edges are the base edges. There may, however, be straight or
curved lines drawn on the surface, and the intersection of the plane with these lines be found,
thereby locating points on the required curved intersection.

7.2.1 INTERSECTION OF SURFACE OF REVOLUTION AND PLANE

Intersection of cylinder and plane
Kinds of intersection shape are presented in Figure 7.4.

64