Page 66 - 4234

a) b)
Figure 7.5

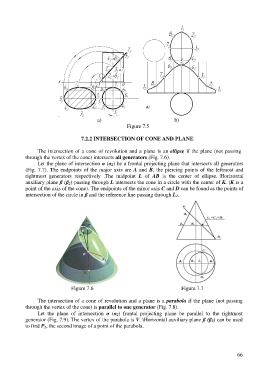
7.2.2 INTERSECTION OF CONE AND PLANE

The intersection of a cone of revolution and a plane is an ellipse if the plane (not passing
through the vertex of the cone) intersects all generators (Fig. 7.6).
Let the plane of intersection α (α 2) be a frontal projecting plane that intersects all generators
(Fig. 7.7). The endpoints of the major axis are A and B, the piercing points of the leftmost and
rightmost generators respectively .The midpoint L of AB is the center of ellipse. Horizontal
auxiliary plane β (β 2) passing through L intersects the cone in a circle with the center of K. (K is a
point of the axis of the cone). The endpoints of the minor axis C and D can be found as the points of
intersection of the circle in β and the reference line passing through L 2.

Figure 7.6 Figure 7.7

The intersection of a cone of revolution and a plane is a parabola if the plane (not passing
through the vertex of the cone) is parallel to one generator (Fig. 7.8).
Let the plane of intersection α (α 2) frontal projecting plane be parallel to the rightmost
generator (Fig. 7.9). The vertex of the parabola is V. \Horizontal auxiliary plane β (β 2) can be used
to find P 2, the second image of a point of the parabola.

66