Page 73 - 4234

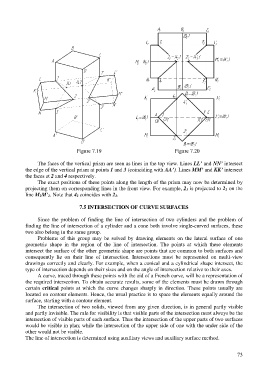
Figure 7.19 Figure 7.20

The faces of the vertical prism are seen as lines in the top view. Lines LL’ and NN’ intersect
the edge of the vertical prism at points 1 and 3 (coinciding with AA’). Lines MM’ and KK’ intersect
the faces at 2 and 4 respectively.
The exact positions of these points along the length of the prism may now be determined by
projecting them on corresponding lines in the front view. For example, 2 1 is projected to 2 2 on the
line M 2M’ 2. Note that 4 2 coincides with 2 2.

7.5 INTERSECTION OF CURVE SURFACES

Since the problem of finding the line of intersection of two cylinders and the problem of
finding the line of intersection of a cylinder and a cone both involve single-curved surfaces, these
two also belong in the same group.
Problems of this group may be solved by drawing elements on the lateral surface of one
geometric shape in the region of the line of intersection. The points at which these elements
intersect the surface of the other geometric shape are points that are common to both surfaces and
consequently lie on their line of intersection. Intersections must be represented on multi-view
drawings correctly and clearly. For example, when a conical and a cylindrical shape intersect, the
type of intersection depends on their sizes and on the angle of intersection relative to their axes.
A curve, traced through these points with the aid of a French curve, will be a representation of
the required intersection. To obtain accurate results, some of the elements must be drawn through
certain critical points at which the curve changes sharply in direction. These points usually are
located on contour elements. Hence, the usual practice is to space the elements equally around the
surface, starting with a contour element.
The intersection of two solids, viewed from any given direction, is in general partly visible
and partly invisible. The rule for visibility is that visible parts of the intersection must always be the
intersection of visible parts of each surface. Thus the intersection of the upper parts of two surfaces
would be visible in plan; while the intersection of the upper side of one with the under side of the
other would not be visible.
The line of intersection is determined using auxiliary views and auxiliary surface method.

73