Page 69 - 4234

As regards the development of the section cut by plane Σ, the different points of the curve
may be found on the elements by laying off from S the true distance from the points of intersection
to the vertex S. The distance S 2F 2 in elevation is a true length, since SF is parallel to Π 2. The point
2 is then located at once. The true distance of the other points from the apex may be found by
projecting horizontally from the points in elevation to S 2F 2, on which line their true distances from
S are shown. These distances are then laid off from S on the respective elements; and a smooth
curve drawn through the points will be the section curve required.
Attention is called to the fact that the length of the developed base I, II, III, … I etc. is not
exact, since, in the first place, it is impossible with the dividers to measure exactly the length of a
curve; and in the second place, because it is likewise impossible to apply exactly with the dividers
any given distance along a curve. It should be said, however, that by taking points sufficiently close
together a very good approximation indeed can be obtained.
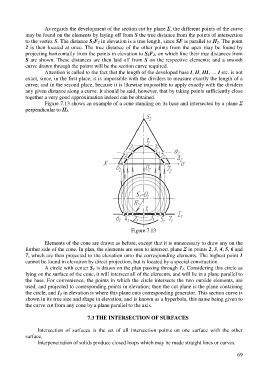
Figure 7.13 shows an example of a cone standing on its base and intersected by a plane Σ
perpendicular to Π 1.

Figure 7.13

Elements of the cone are drawn as before, except that it is unnecessary to draw any on the
further side of the cone. In plan, the elements are seen to intersect plane Σ in points 2, 3, 4, 5, 6 and
7, which are then projected to the elevation onto the corresponding elements. The highest point 1
cannot be found in elevation by direct projection, but is located by a special construction.
A circle with center S 1 is drawn on the plan passing through 1 1. Considering this circle as
lying on the surface of the cone, it will intersect all of the elements, and will lie in a plane parallel to
the base. For convenience, the points in which the circle intersects the two outside elements, are
used, and projected to corresponding points in elevation; then the cut plane is the plane containing
the circle, and 1 2 in elevation is where this plane cuts corresponding generator. This section curve is
shown in its true size and shape in elevation, and is known as a hyperbola, this name being given to
the curve cut from any cone by a plane parallel to the axis.

7.3 THE INTERSECTION OF SURFACES

Intersection of surfaces is the set of all intersection points on one surface with the other
surface.
Interpenetration of solids produce closed loops which may be made straight lines or curves.

69