Page 87 - 4167

forces arising from pressure differences. If every element passing
through a given point follows the same line of flow as that of preceding
elements, the flow is said to be steady or stationary
In steady flow, the velocity at each point of space remains constant

in time, although the velocity of a particular particle of the fluid may
change as it moves from one point to another. A streamline or line of
flow. is defined as a curve whose tangent, at any point, is in the

direction of the fluid velocity at that point as shown in fig 7.1.
If we construct all of the streamlines passing through the periphery
* of an element of area, such as the area A in fig. 7.2, these lines enclose
a tube called a. flow tube or tube of flow. From the definition of a

streamline, no fluid can cross the side walls of a tube of flow; in steady
flow there can be no mixing of the fluids in different flow tubes.

7.2 The Equation of Continuity

Let us consider any stationary, closed surface in a moving fluid; in

general, fluid flows into the volume enclosed by the surface at some
points and flows out at other points. The equation of continuity is a
mathematical statement of the fact that the net rate of flow of mass

inward across any closed surface is equal to the rate of increase of the
mass within the surface.
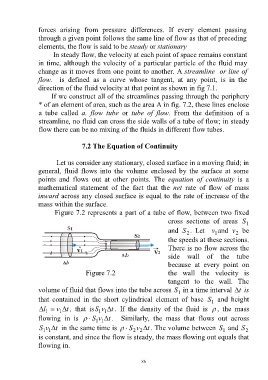
Figure 7.2 represents a part of a tube of flow, between two fixed
cross sections of areas S
1
and S . Let v and v be
2
2
1
the speeds at these sections.
There is no flow across the
side wall of the tube
because at every point on

Figure 7.2 the wall the velocity is
tangent to the wall. The

volume of fluid that flows into the tube across S in a time interval t is
1
that contained in the short cylindrical element of base S and height
1
 l  v 1 t  , that is vS 1 1 t  . If the density of the fluid is  , the mass
1
flowing in is  S  1 v 1 t  . Similarly, the mass that flows out across

S 1 v 1 t  in the same time is  S 2 v 2 t  . The volume between S and S
2
1
is constant, and since the flow is steady, the mass flowing out equals that
flowing in.

86