Page 84 - 4167

mg  r 
  (6.45)
L
take into account that  IL  

mg   r
  . (6.46)
 I 
Thereby we deduced formula of angular velocity of precession
Gyroscopic precession also plays a large role in the flight controls
on helicopters. Since the driving force behind helicopters is the rotor

disk (which rotates), gyroscopic precession comes into play. If the rotor
disk tilted forward (to gain forward velocity), its rotation requires that
the downward net force on the blade be applied roughly 90 degrees

(depending on blade configuration) before, or when the blade is to one
side of the pilot and rotating
forward.
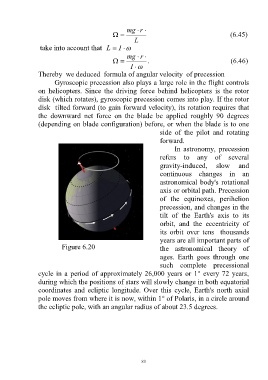
In astronomy, precession

refers to any of several
gravity-induced, slow and
continuous changes in an

astronomical body's rotational
axis or orbital path. Precession
of the equinoxes, perihelion

precession, and changes in the
tilt of the Earth's axis to its
orbit, and the eccentricity of

its orbit over tens thousands
years are all important parts of
Figure 6.20 the astronomical theory of
ages. Earth goes through one

such complete precessional
cycle in a period of approximately 26,000 years or 1° every 72 years,
during which the positions of stars will slowly change in both equatorial

coordinates and ecliptic longitude. Over this cycle, Earth's north axial
pole moves from where it is now, within 1° of Polaris, in a circle around
the ecliptic pole, with an angular radius of about 23.5 degrees.

83