Page 90 - 4167

In this form, Bernoulli’s equation represents the equality of the
work per unit volume of fluid to the sum of the changes in kinetic and
potential energies per unit volume that occur during the flow. Or we
may interpret Eq. (7.7) in terms of pressures. The second term on the

right is the pressure difference arising from the weight of the fluid and
the difference in elevation of the two ends of the fluid element. The first
term on the right is the additional pressure difference associated with the

change of velocity of the fluid. equation (7.7) can also be written
2
 v  1 2  v  2
p    g  h   p    g  h  (7.9)
2
2
1
1
2 2
and since the subscripts 1 and 2 refer to any two points along the tube of
flow, Bernoulli's equation may also be written
2
 v 
p    g  h   const (7.10)
2
2
 v 
where p is static pressure,  g h  -hydrostatic pressure, ,
2

dynamic pressure,
7.4 Applications of Bernoulli's Equation

1. The equations of hydrostatics are special cases of Bernoulli's
equation, when the velocity is zero everywhere. Thus, when v and v are
2
1
zero, eq. (7.7) reduce to
p  p   g  (h  h 1 ) . (7.10)
2
1
2

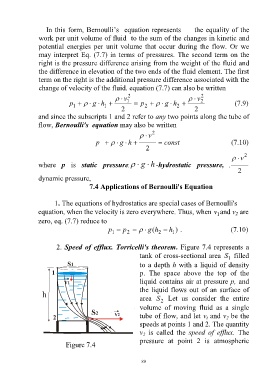
2. Speed of efflux. Torricelli's theorem. Figure 7.4 represents a
tank of cross-sectional area S filled
1
to a depth h with a liquid of density
p. The space above the top of the
liquid contains air at pressure p, and

the liquid flows out of an surface of
area S Let us consider the entire
2
volume of moving fluid as a single

tube of flow, and let v and v be the
2
t
speeds at points 1 and 2. The quantity
v is called the speed of efflux. The
2
pressure at point 2 is atmospheric
Figure 7.4

89