Page 83 - 4167

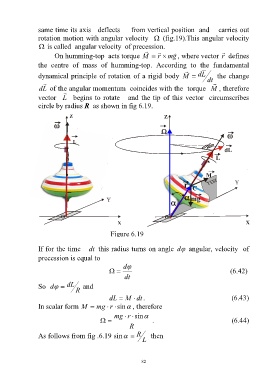
same time its axis deflects from vertical position and carries out
rotation motion with angular velocity  (fig.19).This angular velocity
 is called angular velocity of precession.
   
On humming-top acts torque M  r  m g , where vector r defines
the centre of mass of humming-top. According to the fundamental

dynamical principle of rotation of a rigid body M  d L the change
 dt 
d L of the angular momentum coincides with the torque M , therefore

vector L begins to rotate and the tip of this vector circumscribes
circle by radius R as shown in fig 6.19.

Figure 6.19

If for the time dt this radius turns on angle d angular, velocity of

precession is equal to
d
  (6.42)
dt

So d  dL and
R
dL  M  dt. (6.43)
In scalar form M  mg   r sin  , therefore

mg  r sin 
  . (6.44)
R
As follows from fig .6.19 sin   R then
L

82