Page 91 - 4167

p . Applying Bernoulli's equation to points 1 and 2, and taking the
a
bottom of the tank as our reference level, we get

2
 v  1 2  v  2
p    pa  (7.11)
2 2
or
p  p a
2
2
v  v  2  2 gh (7.12)
1
2
p

Now let us consider some special cases. Suppose , that

2
2
S S   Then v is much less than v and can be neglected. Suppose
2 1 1 2
also that p  p therefore
a
v  2 g  h  . (7.13)
2
That is, the speed of efflux is the same as the speed a body would
acquire in falling freely from a height h. This is Torricelli's theorem.
It is not restricted to an opening in the bottom of a vessel, but applied
also to a hole in the side walls at a depth h below the surface.

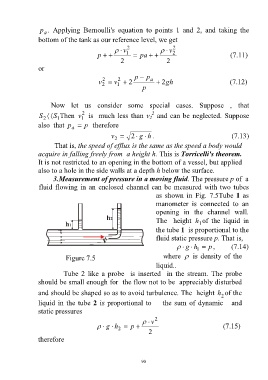
3.Measurement of pressure in a moving fluid. The pressure p of a
fluid flowing in an enclosed channel can be measured with two tubes
as shown in Fig. 7.5Tube 1 as

manometer is connected to an
opening in the channel wall.
The height h of the liquid in
1
the tube 1 is proportional to the
fluid static pressure p. That is,

 g  h  p, (7.14)
1
Figure 7.5 where  is density of the
liquid..
Tube 2 like a probe is inserted in the stream. The probe
should be small enough for the flow not to be appreciably disturbed

and should be shaped so as to avoid turbulence. The height h of the
2
liquid in the tube 2 is proportional to the sum of dynamic and
static pressures

2
 v 
 g  h  p  (7.15)
2
2
therefore

90