Page 88 - 4167

Hence
 S 1 v  t   S  2 v 2 t  , (7.1)
1
or

vS 1 1  S  2 v . (7.2)
2

v
Thereby, the product S  is constant along any-given tube of
flow and it is equation of continuity. It follows that when the cross
section of a flow tube decreases, as in the constriction in Fig.7.2, the
velocity increases. This can readily be shown by introducing small

particles into the fluid and observing their motion.
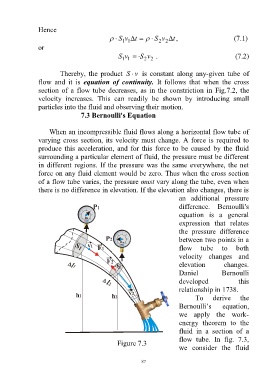
7.3 Bernoulli's Equation

When an incompressible fluid flows along a horizontal flow tube of
varying cross section, its velocity must change. A force is required to
produce this acceleration, and for this force to be caused by the fluid

surrounding a particular element of fluid, the pressure must be different
in different regions. If the pressure was the same everywhere, the net
force on any fluid element would be zero. Thus when the cross section

of a flow tube varies, the pressure must vary along the tube, even when
there is no difference in elevation. If the elevation also changes, there is
an additional pressure
difference. Bernoulli's

equation is a general
expression that relates
the pressure difference

between two points in a
flow tube to both
velocity changes and
elevation changes.

Daniel Bernoulli
developed this
relationship in 1738.

To derive the
Bernoulli’s equation,
we apply the work-

energy theorem to the
fluid in a section of a
flow tube. In fig. 7.3,
Figure 7.3
we consider the fluid

87