Page 85 - 4167

6.8Questions

1.How to deduce the formula of kinetics energy of rotational motion

of rigid body ?
2. Why moment of inertia is analogous to mass m ?
3.How can we calculate the moment of inertia of different rigid bodies?

4.What does the main law of dynamic of rotational motion state?
5.What's angular momentum?
6. What does law of conservation of angular momentum states ?
7.Give examples of conservation of angular momentum.

8. What are free axes of rotation?
9. What's tensor of inertia?
10 What's gyroscope and its properties?

6.9.Problems

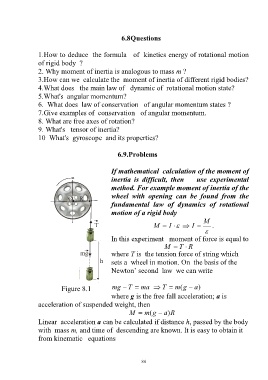
If mathematical calculation of the moment of
inertia is difficult, then use experimental
method. For example moment of inertia of the

wheel with opening can be found from the
fundamental law of dynamics of rotational
motion of a rigid body
M
M  I    I  .

In this experiment moment of force is equal to

M  T  R
where T is the tension force of string which
sets a wheel in motion. On the basis of the
Newton’ second law we can write

Figure 8.1 mg  T  ma  T  m (g  ) a
where g is the free fall acceleration; a is

acceleration of suspended weight, then
M  m( g  a) R
Linear acceleration a can be calculated if distance h, passed by the body

with mass m, and time of descending are known. It is easy to obtain it
from kinematic equations

84