Page 78 - 4167

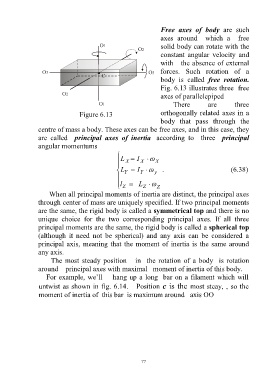
Free axes of body are such
axes around which a free
solid body can rotate with the
constant angular velocity and

with the absence of external
forces. Such rotation of a
body is called free rotation.

Fig. 6.13 illustrates three free
axes of parallelepiped
There are three

Figure 6.13 orthogonally related axes in a
body that pass through the
centre of mass a body. These axes can be free axes, and in this case, they
are called principal axes of inertia according to three principal

angular momentums
 L  I 
 X X X

L  I Y  y . (6.38)
 Y

l  L Z  Z
 Z
When all principal moments of inertia are distinct, the principal axes
through center of mass are uniquely specified. If two principal moments

are the same, the rigid body is called a symmetrical top and there is no
unique choice for the two corresponding principal axes. If all three
principal moments are the same, the rigid body is called a spherical top
(although it need not be spherical) and any axis can be considered a

principal axis, meaning that the moment of inertia is the same around
any axis.
The most steady position in the rotation of a body is rotation

around principal axes with maximal moment of inertia of this body.
For example, we’ll hang up a long bar on a filament which will
untwist as shown in fig. 6.14. Position c is the most steay, , so the

moment of inertia of this bar is maximum around axis OO

77