Page 61 - 6637

Supplementary Problems

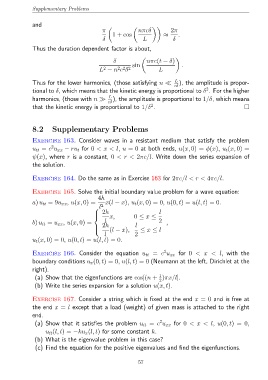
and

π nπcδ 2π
1 + cos ≈ .
δ L δ
Thus the duration dependent factor is about,

δ nπc(t − δ)
sin .
2 2 2
2
L − n c δ L
Thus for the lower harmonics, (those satisfying n L ), the amplitude is propor-
cδ
2
tional to δ, which means that the kinetic energy is proportional to δ . For the higher
harmonics, (those with n L ), the amplitude is proportional to 1/δ, which means
cδ
2
that the kinetic energy is proportional to 1/δ .

8.2 Supplementary Problems

Exercise 163. Consider waves in a resistant medium that satisfy the problem
2
u = c u xx − ru for 0 < x < l, u = 0 at both ends, u(x, 0) = φ(x), u (x, 0) =
tt
t
t
ψ(x), where r is a constant, 0 < r < 2πc/l. Write down the series expansion of
the solution.

Exercise 164. Do the same as in Exercise 163 for 2πc/l < r < 4πc/l.

Exercise 165. Solve the initial boundary value problem for a wave equation:
4h
a) u = 9u , u(x, 0) = x(l − x), u (x, 0) = 0, u(0, t) = u(l, t) = 0.
xx
t
tt
l 2

2h l
x, 0 ≤ x ≤


l
b) u = u , u(x, 0) = 2h l 2 ,
xx
tt
 (l − x), ≤ x ≤ l

l 2
u (x, 0) = 0, u(0, t) = u(l, t) = 0.
t
2
Exercise 166. Consider the equation u tt = c u xx for 0 < x < l, with the
boundary conditions u (0, t) = 0, u(l, t) = 0 (Neumann at the left, Dirichlet at the
x
right).
1
(a) Show that the eigenfunctions are cos[(n + )πx/l].
2
(b) Write the series expansion for a solution u(x, t).
Exercise 167. Consider a string which is fixed at the end x = 0 and is free at
the end x = l except that a load (weight) of given mass is attached to the right

end.
2
(a) Show that it satisfies the problem u = c u xx for 0 < x < l, u(0, t) = 0,
tt
u (l, t) = −ku (l, t) for some constant k.
x
tt
(b) What is the eigenvalue problem in this case?
(c) Find the equation for the positive eigenvalues and find the eigenfunctions.

57