Page 60 - 6637

Solved Problems

2
3
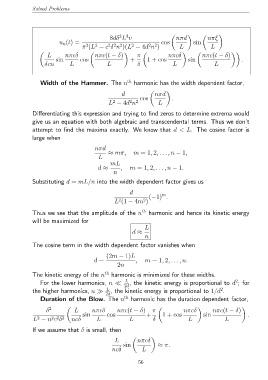
8dδ L v nπd nπξ
u (t) = cos sin
n
2 2
3
2
2 2 2
2
π (L − c δ n )(L − 4d n ) L L

L nπcδ nπc(t − δ) π nπcδ nπc(t − δ)
sin cos + 1 + cos sin .
δcn L L δ L L
Width of the Hammer. The n th harmonic has the width dependent factor,

d nπd
cos .
2 2
2
L − 4d n L
Differentiating this expression and trying to find zeros to determine extrema would
give us an equation with both algebraic and transcendental terms. Thus we don’t
attempt to find the maxima exactly. We know that d < L. The cosine factor is

large when
nπd
≈ mπ, m = 1, 2, . . . , n − 1,
L
mL
d ≈ , m = 1, 2, . . . , n − 1.
n

Substituting d = mL/n into the width dependent factor gives us

d
m
(−1) .
2
2
L (1 − 4m )
Thus we see that the amplitude of the n th harmonic and hence its kinetic energy
will be maximized for
L
d ≈
n
The cosine term in the width dependent factor vanishes when

(2m − 1)L
d = , m = 1, 2, . . . , n.
2n
The kinetic energy of the n th harmonic is minimized for these widths.
2
For the lower harmonics, n L , the kinetic energy is proportional to d ; for
2d
2
the higher harmonics, n L , the kinetic energy is proportional to 1/d .
2d
Duration of the Blow. The n th harmonic has the duration dependent factor,
δ 2 L nπcδ nπc(t − δ) π nπcδ nπc(t − δ)
sin cos + 1 + cos sin .
2 2 2
2
L − n c δ ncδ L L δ L L
If we assume that δ is small, then

L nπcδ
sin ≈ π.
ncδ L

56