Page 65 - 6637

Solved Problems

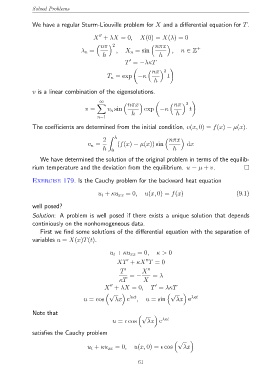
We have a regular Sturm-Liouville problem for X and a differential equation for T.

00
X + λX = 0, X(0) = X(λ) = 0
nπ nπx
2
λ = , X = sin , n ∈ Z +
n
n
h h
0
T = −λκT

nπ
2
T = exp −κ t
n
h
v is a linear combination of the eigensolutions.
∞
2
X nπx nπ
v = v sin exp −κ t
n
h h
n=1
The coefficients are determined from the initial condition, v(x, 0) = f(x) − µ(x).

Z h
2 nπx
v = (f(x) − µ(x)) sin dx
n
h 0 h
We have determined the solution of the original problem in terms of the equilib-
rium temperature and the deviation from the equilibrium. u = µ + v.

Exercise 179. Is the Cauchy problem for the backward heat equation

u + κu xx = 0, u(x, 0) = f(x) (9.1)
t
well posed?

Solution: A problem is well posed if there exists a unique solution that depends
continiously on the nonhomogeneous data.

First we find some solutions of the differential equation with the separation of
variables u = X(x)T(t).

u + κu xx = 0, κ > 0
t
0
00
XT + κX T = 0
T 0 X 00
= − = λ
κT X
0
00
X + λX = 0, T = λκT
√ √

u = cos λx e λκt , u = sin λx e λκt

Note that
√
u = cos λx e λκt

satisfies the Cauchy problem
√

u + κu xx = 0, u(x, 0) = cos λx
t

61