Page 28 - 4811

Laplace Transforms of Periodic Functions

The Laplace transform of a T-periodic function is given next.

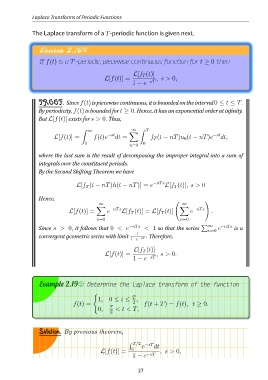
Theorem 2.10È

If f(t) is a T-periodic, piecewise continuous function for t ≥ 0 then

L[f (t)]
T
L[f(t)] = , s > 0,
1 − e −sT
. . . . . . .

PROOF. Sincef(t)ispiecewisecontinuous,itisboundedontheinterval0 ≤ t ≤ T.
By periodicity, f(t) is bounded for t ≥ 0. Hence, it has an exponential order at infinity.
But L[f(t)] exists for s > 0. Thus,

∫ ∞ ∫
∞ T
∑
L[f(t)] = f(t)e −st dt = f (t − nT)u (t − nT)e −st dt,
0
T
0 n=0 0
where the last sum is the result of decomposing the improper integral into a sum of
integrals over the constituent periods.

By the Second Shifting Theorem we have

L[f (t − nT)h(t − nT)] = e −nTs L[f (t)], s > 0
T
T
Hence,
( )
∞ ∞
∑ ∑
L[f(t)] = e −nTs L[f (t)] = L[f (t)] e −nTs .
T
T
n=0 n=0
∑ ∞ −nTs
−nTs
Since s > 0, it follows that 0 < e < 1 so that the series e is a
n=0
convergent geometric series with limit 1 . Therefore,
1−e −sT
L[f (t)]
T
L[f(t)] = , s > 0.
1 − e −sT
. . . .
.

Example 2.19, Determine the Laplace transform of the function

{
T
1, 0 ≤ t ≤ ,
f(t) = 2 f(t + T) = f(t), t ≥ 0.
0, T < t < T,
2
. . . . .
.

Solution. By previous theorem,

T/2 e −sT dt
∫
L[f(t)] = 0 , s > 0,
. . . . 1 − e −sT

27