Page 54 - 4749

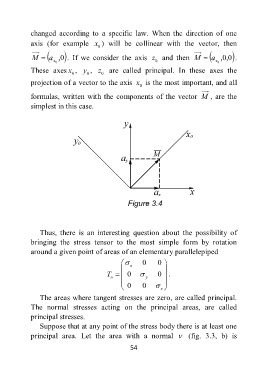
changed according to a specific law. When the direction of one
axis (for example x ) will be collinear with the vector, then
0
M  a  0 , . If we consider the axis z and then M  a  0 , 0 , .
0 x 0 0 x
These axes x , y , z are called principal. In these axes the
0 0 0
projection of a vector to the axis x is the most important, and all
0
formulas, written with the components of the vector M , are the
simplest in this case.

Figure 3.4

Thus, there is an interesting question about the possibility of
bringing the stress tensor to the most simple form by rotation
around a given point of areas of an elementary parallelepiped
  x 0 0 
 
T  0  0 .
н  y 
 0 0  
 z 
The areas where tangent stresses are zero, are called principal.
The normal stresses acting on the principal areas, are called
principal stresses.
Suppose that at any point of the stress body there is at least one
principal area. Let the area with a normal  (fig. 3.3, b) is
54