Page 57 - 4749

  i 0 0    i 0 0   i 0 0
     
T  0  0 T  0  0 T  0 0 0
н  j  н  j  н  
 0 0    0 0 0   0 0 0 
 k     

i , j , k 3 , 2 , 1 , i j  1,2,3 i  1,3
 i  i  i
 k

 j  j  j  j
 k
 i  i  i

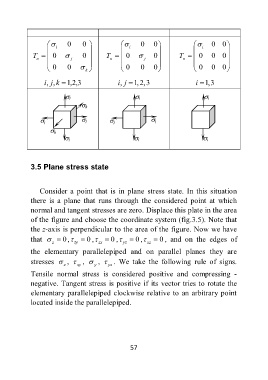
3.5 Plane stress state

Consider a point that is in plane stress state. In this situation
there is a plane that runs through the considered point at which
normal and tangent stresses are zero. Displace this plate in the area
of the figure and choose the coordinate system (fig.3.5). Note that
the z-axis is perpendicular to the area of the figure. Now we have
that   0,  0 ,  0 ,  0 ,  0 , and on the edges of
z zy zx yz xz
the elementary parallelepiped and on parallel planes they are
stresses  ,  ,  ,  . We take the following rule of signs.
x xy y yx
Tensile normal stress is considered positive and compressing -
negative. Tangent stress is positive if its vector tries to rotate the
elementary parallelepiped clockwise relative to an arbitrary point
located inside the parallelepiped.

57