Page 58 - 4749

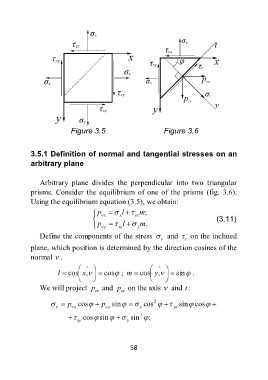
Figure 3.5 Figure 3.6

3.5.1 Definition of normal and tangential stresses on an
arbitrary plane

Arbitrary plane divides the perpendicular into two triangular
prisms. Consider the equilibrium of one of the prisms (fig. 3.6).
Using the equilibrium equation (3.5), we obtain:
 p   x l  yx ; m

x
 (3.11)
 p   xy l  y . m

y
Define the components of the stress  and  on the inclined
 t
plane, which position is determined by the direction cosines of the
normal  .
     
l cos x  ,   cos  ; m  cos y  ,   sin  .
   
We will project p and p on the axis  and t :
x  y 
2
  p cos  p sin   cos   sin cos  
  x  y x yx

2
 cos sin   sin  ;
xy y
58