Page 51 - 4749

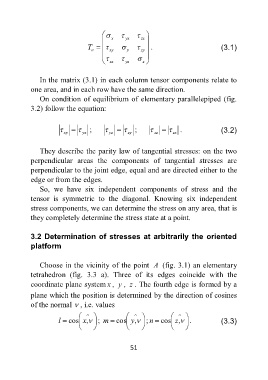
  x  yx  
zx
 
T     . (3.1)
н  xy y zy 
    
 xz yz z 

In the matrix (3.1) in each column tensor components relate to
one area, and in each row have the same direction.
On condition of equilibrium of elementary parallelepiped (fig.
3.2) follow the equation:

   ;    ;    . (3.2)
xy yx yz zy xz zx

They describe the parity law of tangential stresses: on the two
perpendicular areas the components of tangential stresses are
perpendicular to the joint edge, equal and are directed either to the
edge or from the edges.
So, we have six independent components of stress and the
tensor is symmetric to the diagonal. Knowing six independent
stress components, we can determine the stress on any area, that is
they completely determine the stress state at a point.

3.2 Determination of stresses at arbitrarily the oriented
platform

Choose in the vicinity of the point A (fig. 3.1) an elementary
tetrahedron (fig. 3.3 a). Three of its edges coincide with the
coordinate plane system x , y , z . The fourth edge is formed by a
plane which the position is determined by the direction of cosines
of the normal  , i.e. values
        
 cosl  ,x  ; m  cos  ,y  ;  cosn  ,z  . (3.3)
     

51