Page 50 - 4749

Through each point of a loaded body we can draw a lot
differently oriented areas. The combination of stresses arising in
these areas, characterizes the state of stress at a point.
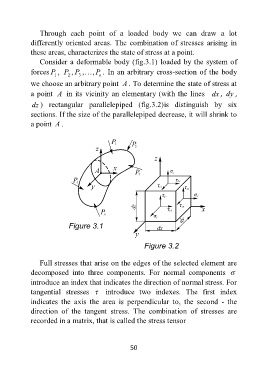
Consider a deformable body (fig.3.1) loaded by the system of
forces P , P , P , ,P . In an arbitrary cross-section of the body
1 2 3 n
we choose an arbitrary point A . To determine the state of stress at
a point A in its vicinity an elementary (with the lines dx , dy ,
dz ) rectangular parallelepiped (fig.3.2)is distinguish by six
sections. If the size of the parallelepiped decrease, it will shrink to
a point A .

Figure 3.1

Figure 3.2

Full stresses that arise on the edges of the selected element are
decomposed into three components. For normal components 
introduce an index that indicates the direction of normal stress. For
tangential stresses  introduce two indexes. The first index
indicates the axis the area is perpendicular to, the second - the
direction of the tangent stress. The combination of stresses are
recorded in a matrix, that is called the stress tensor

50