Page 52 - 4749

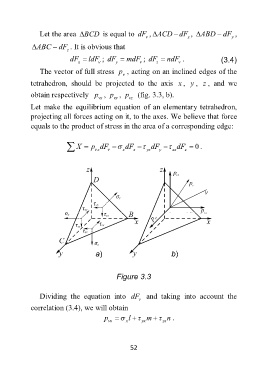
Let the area BCD is equal to dF , ACD  dF , ABD  dF ,
 x y
 ABC  dF . It is obvious that
z
dF  ldF ; dF  mdF ; dF  ndF . (3.4)
x  y  z 
The vector of full stress p , acting on an inclined edges of the

tetrahedron, should be projected to the axis x , y , z , and we
obtain respectively p , p , p (fig. 3.3, b).
x  y  z 
Let make the equilibrium equation of an elementary tetrahedron,
projecting all forces acting on it, to the axes. We believe that force
equals to the product of stress in the area of a corresponding edge:

 X  p dF   x dF   yx dF   zx dF  0 .

z
x
y

x

a) b)

Figure 3.3

Dividing the equation into dF and taking into account the

correlation (3.4), we will obtain
p   l  m  n .
x  x yx zx

52