Page 35 - 4749

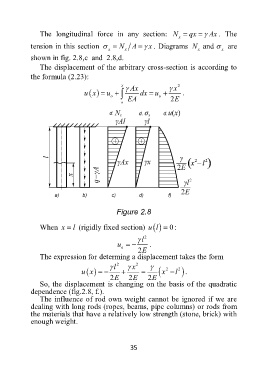
The longitudinal force in any section: N  qx  Ax . The
x
tension in this section   N A  x . Diagrams N and  are
x x x x
shown in fig. 2.8,c and 2.8,d.
The displacement of the arbitrary cross-section is according to
the formula (2.23):
x  Ax  x 2
o 
u   x  u  dx  u  .
o
o EA 2E

Figure 2.8

u
0
When x  (rigidly fixed section)   l  :
l
l  2
u   .
o
2E
The expression for determing a displacement takes the form
l  2  x 2 
2
u   x      x  l 2  .
2E 2E 2E
So, the displacement is changing on the basis of the quadratic
dependence (fig.2.8, f.).
The influence of rod own weight cannot be ignored if we are
dealing with long rods (ropes, beams, pipe columns) or rods from
the materials that have a relatively low strength (stone, brick) with
enough weight.

35