Page 40 - 4749

Usually during practical calculations for brittle materials the
deviation from Hooke's law does not take into account. In this case
the concept of sectional elasticity modulus E is introduced, that
S
equals the tangent of slope angle of the line drawn across diagram
starting point O and a point A:


E  tg  A .
S

A
Sectional module - a variable value, it can be considered a
function of stress. To calculate the structural elements of brittle
material the average value of the elastic sectional modulus is taken
that corresponds to the expected range of operating pressure.

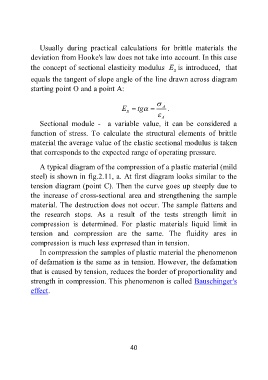
A typical diagram of the compression of a plastic material (mild
steel) is shown in fig.2.11, a. At first diagram looks similar to the
tension diagram (point C). Then the curve goes up steeply due to
the increase of cross-sectional area and strengthening the sample
material. The destruction does not occur. The sample flattens and
the research stops. As a result of the tests strength limit in
compression is determined. For plastic materials liquid limit in
tension and compression are the same. The fluidity ares in
compression is much less exprresed than in tension.
In compression the samples of plastic material the phenomenon
of defamation is the same as in tension. However, the defamation
that is caused by tension, reduces the border of proportionality and
strength in compression. This phenomenon is called Bauschinger's
effect.

40