Page 33 - 4749

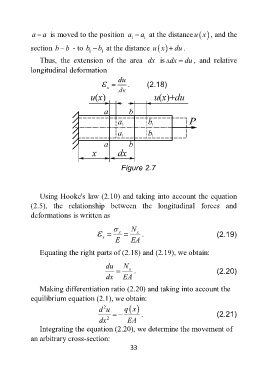
a a is moved to the position a  a at the distance   x , and the
u
1 1
b
u
section b b - to b  at the distance   x  du .
1 1
Thus, the extension of the area dx is dx  du , and relative
longitudinal deformation
du
  . (2.18)
x
dx

Figure 2.7

Using Hooke's law (2.10) and taking into account the equation
(2.5), the relationship between the longitudinal forces and
deformations is written as
 N
  x  x . (2.19)
x
E EA
Equating the right parts of (2.18) and (2.19), we obtain:

du N
 x . (2.20)
dx EA
Making differentiation ratio (2.20) and taking into account the
equilibrium equation (2.1), we obtain:
2
d u q   x
  . (2.21)
dx 2 EA
Integrating the equation (2.20), we determine the movement of
an arbitrary cross-section:
33