Page 31 - 4749

numerically equal to tension that arises in the rod while increasing
its length twice as large.
The result of the multiplication EA is called cross-section
stiffness in tension and compression. This is a complex physical
and geometrical characteristic of the rod. This module E describes
the physical properties of the material, and the area A describes
the geometric properties of the rod.
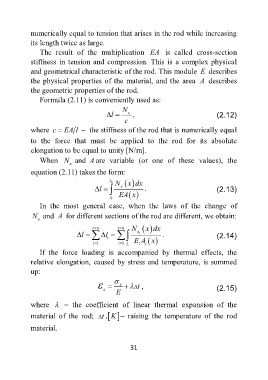
Formula (2.11) is conveniently used as:
N
l   x , (2.12)
c
where c  EA l – the stiffness of the rod that is numerically equal
to the force that must be applied to the rod for its absolute
elongation to be equal to unity [N/m].
When N and A are variable (or one of these values), the
x
equation (2.11) takes the form:
0 l N   x dx
l    x . (2.13)
0 EA   x
In the most general case, when the laws of the change of
N and A for different sections of the rod are different, we obtain:
x
i n i n N   x dx
i 
l    l   i x . (2.14)
i 1 i 1 l E A i   x
i
i
If the force loading is accompanied by thermal effects, the
relative elongation, caused by stress and temperature, is summed
up:

  x   t ,
x (2.15)
E
where  – the coefficient of linear thermal expansion of the
material of the rod; t , – raising the temperature of the rod
K
material.

31