Page 99 - 4624

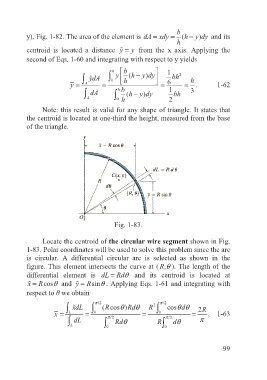
b
y), Fig. 1-82. The area of the element is dA = xdy = (h − ) y dy and its
h
y
ɶ
centroid is located a distance y = from the x axis. Applying the
second of Eqs. 1-60 and integrating with respect to y yields
h b   1
ydA ∫ɶ 0  y  ∫ h (h − ) y dy  bh 2 h
y = A = h b  = 6 = . 1-62
∫ A dA ∫ 0 h (h − ) y dy 1 bh 3
2
Note: this result is valid for any shape of triangle. It states that
the centroid is located at one-third the height, measured from the base
of the triangle.

Fig. 1-83.

Locate the centroid of the circular wire segment shown in Fig.
1-83. Polar coordinates will be used to solve this problem since the arc
is circular. A differential circular arc is selected as shown in the
figure. This element intersects the curve at ( ,R θ ). The length of the
differential element is dL = Rdθ and its centroid is located at
x = R cosθ and y = R sinθ . Applying Eqs. 1-61 and integrating with
ɶ
ɶ
respect to θ we obtain
∫ xdL ∫ π /2 ( cos )R θ Rdθ R 2 ∫ π /2 cos dθθ 2R
ɶ
x = L = 0 = 0 = , 1-63
∫ L dL ∫ 0 π /2 Rdθ R ∫ 0 π /2 dθ π

99