Page 100 - 4624

∫ ydL ∫ π /2 ( sin )R θ Rdθ R 2 ∫ π /2 sin dθθ 2R
ɶ
y = L = 0 = 0 = . 1-64
∫ L dL ∫ 0 π /2 Rdθ R ∫ 0 π /2 dθ π

Note: As expected, the two coordinates are numerically the same
due to the symmetry of the wire.
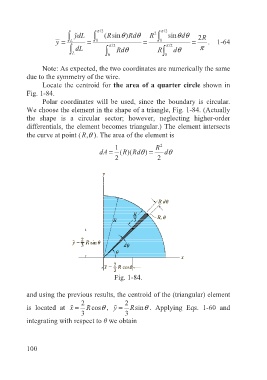
Locate the centroid for the area of a quarter circle shown in
Fig. 1-84.
Polar coordinates will be used, since the boundary is circular.
We choose the element in the shape of a triangle, Fig. 1-84. (Actually
the shape is a circular sector; however, neglecting higher-order
differentials, the element becomes triangular.) The element intersects
the curve at point ( ,R θ ). The area of the element is
1 R 2
dA = ( )(Rdθ = dθ
)
R
2 2

Fig. 1-84.

and using the previous results, the centroid of the (triangular) element
2 2
is located at x = R cosθ , y = R sinθ . Applying Eqs. 1-60 and
ɶ
ɶ
3 3
integrating with respect to θ we obtain

100