Page 94 - 4624

35 Center of Gravity, Center of Mass, and the Centroid of a
Body

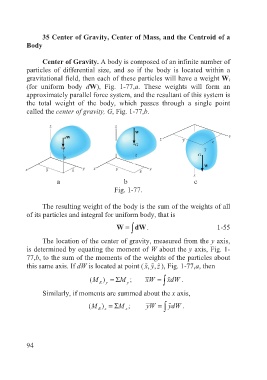
Center of Gravity. A body is composed of an infinite number of
particles of differential size, and so if the body is located within a
gravitational field, then each of these particles will have a weight Wi
(for uniform body dW), Fig. 1-77,a. These weights will form an
approximately parallel force system, and the resultant of this system is
the total weight of the body, which passes through a single point
called the center of gravity, G, Fig. 1-77,b.

a b c
Fig. 1-77.

The resulting weight of the body is the sum of the weights of all
of its particles and integral for uniform body, that is
∫
W = dW. 1-55
The location of the center of gravity, measured from the y axis,
is determined by equating the moment of W about the y axis, Fig. 1-
77,b, to the sum of the moments of the weights of the particles about
this same axis. If dW is located at point ( ,,x yz ɶ ), Fig. 1-77,a, then
ɶɶ
∫
xdW .
(M Ry M ; xW = ɶ
) =Σ
y
Similarly, if moments are summed about the x axis,
∫
(M Rx M ; yW = ɶ
) =Σ
ydW .
x

94