Page 97 - 4624

∫ xdS ∫ ydS ∫ zdS
ɶ
ɶ
ɶ
x = , y = , z = . 1-60
∫ dS ∫ dS ∫ dS

a b c
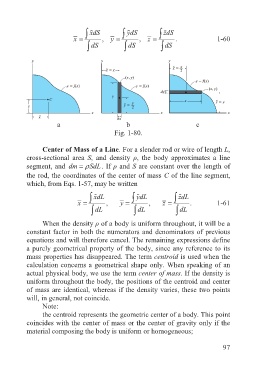
Fig. 1-80.

Center of Mass of a Line. For a slender rod or wire of length L,
cross-sectional area S, and density ρ, the body approximates a line
segment, and dm ρ= SdL. If ρ and S are constant over the length of
the rod, the coordinates of the center of mass C of the line segment,
which, from Eqs. 1-57, may be written
∫ xdL ∫ ydL ∫ zdL
ɶ
ɶ
ɶ
x = , y = , z = . 1-61
∫ dL ∫ dL ∫ dL
When the density ρ of a body is uniform throughout, it will be a
constant factor in both the numerators and denominators of previous
equations and will therefore cancel. The remaining expressions define
a purely geometrical property of the body, since any reference to its
mass properties has disappeared. The term centroid is used when the
calculation concerns a geometrical shape only. When speaking of an
actual physical body, we use the term center of mass. If the density is
uniform throughout the body, the positions of the centroid and center
of mass are identical, whereas if the density varies, these two points
will, in general, not coincide.
Note:
the centroid represents the geometric center of a body. This point
coincides with the center of mass or the center of gravity only if the
material composing the body is uniform or homogeneous;

97