Page 104 - 4624

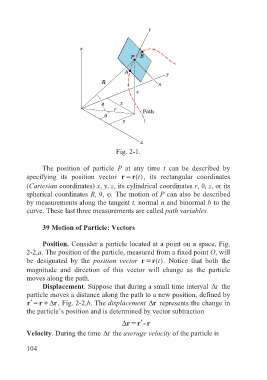
Fig. 2-1.

The position of particle P at any time t can be described by
specifying its position vector r = r ()t , its rectangular coordinates
(Cartesian coordinates) x, y, z, its cylindrical coordinates r, θ, z, or its
spherical coordinates R, θ, φ. The motion of P can also be described
by measurements along the tangent t, normal n and binormal b to the
curve. These last three measurements are called path variables.

39 Motion of Particle: Vectors

Position. Consider a particle located at a point on a space, Fig.
2-2,a. The position of the particle, measured from a fixed point O, will
be designated by the position vector r =r ()t . Notice that both the
magnitude and direction of this vector will change as the particle
moves along the path.
Displacement. Suppose that during a small time interval t∆ the
particle moves a distance along the path to a new position, defined by
′ r =r + ∆r, Fig. 2-2,b. The displacement ∆r represents the change in
the particle’s position and is determined by vector subtraction
∆r = ′ r -r
Velocity. During the time t∆ the average velocity of the particle is

104