Page 101 - 4624


xdA ∫ π /2 2 R cosθ   R 2 dθ 2 R ∫ π /2 cos d
θθ
 ∫
 ɶ
x = A = 0 3  2 = 3 0 = 4R , 1-65
∫ A dA ∫ 0 π /2 R 2 2 dθ ∫ 0 π /2 dθ 3π

θθ
∫ ydA ∫ 0 π /2 2 R sinθ   R 2 2 dθ 2 R ∫ π /2 sin d 4R
 ɶ
3
y = A =   = 3 0 = . 1-66
∫ A dA ∫ 0 π /2 R 2 2 dθ ∫ 0 π /2 dθ 3π

37 Ways of Determination Location the Center of Gravity

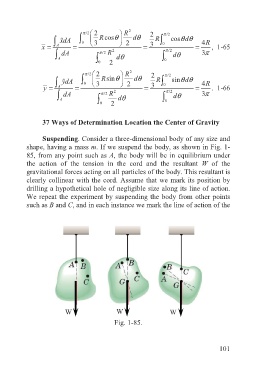
Suspending. Consider a three-dimensional body of any size and
shape, having a mass m. If we suspend the body, as shown in Fig. 1-
85, from any point such as A, the body will be in equilibrium under
the action of the tension in the cord and the resultant W of the
gravitational forces acting on all particles of the body. This resultant is
clearly collinear with the cord. Assume that we mark its position by
drilling a hypothetical hole of negligible size along its line of action.
We repeat the experiment by suspending the body from other points
such as B and C, and in each instance we mark the line of action of the

W W W
Fig. 1-85.

101