Page 150 - 4624

a

b
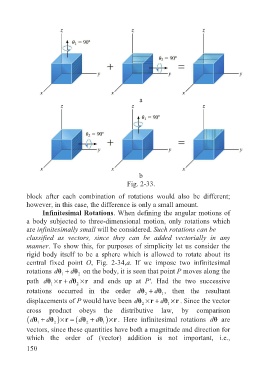
Fig. 2-33.

block after each combination of rotations would also be different;
however, in this case, the difference is only a small amount.
Infinitesimal Rotations. When defining the angular motions of
a body subjected to three-dimensional motion, only rotations which
are infinitesimally small will be considered. Such rotations can be
classified as vectors, since they can be added vectorially in any
manner. To show this, for purposes of simplicity let us consider the
rigid body itself to be a sphere which is allowed to rotate about its
central fixed point O, Fig. 2-34,a. If we impose two infinitesimal
rotations dθ 1 + dθ 2 on the body, it is seen that point P moves along the
path d ×+θ 1 r dθ 2 ×r and ends up at P'. Had the two successive
rotations occurred in the order dθ 2 + dθ 1 , then the resultant
displacements of P would have been dθ 2 ×+r d ×θ 1 r . Since the vector
cross product obeys the distributive law, by comparison
(dθ 1 + dθ 2 )×=r (dθ 2 + dθ 1 )×r . Here infinitesimal rotations dθ are
vectors, since these quantities have both a magnitude and direction for
which the order of (vector) addition is not important, i.e.,
150