Page 148 - 4624

Since the relative-acceleration components represent the effect
of circular motion observed from translating axes having their origin
) =×α r
at the base point A, these terms can be expressed as (a / BA t / B A
2
) =−
and (a / BA n ω r / BA , Eq. 2-63. Hence, Eq. 2-86 becomes

a b
Fig. 2-31.

2
α
a B = a A +×r / BA − ω r / B A . 2-87
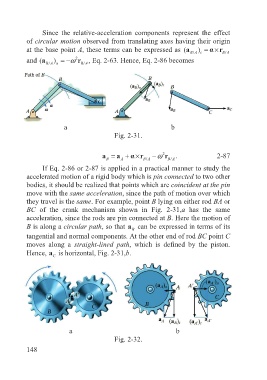
If Eq. 2-86 or 2-87 is applied in a practical manner to study the
accelerated motion of a rigid body which is pin connected to two other
bodies, it should be realized that points which are coincident at the pin
move with the same acceleration, since the path of motion over which
they travel is the same. For example, point B lying on either rod BA or
BC of the crank mechanism shown in Fig. 2-31,a has the same
acceleration, since the rods are pin connected at B. Here the motion of
B is along a circular path, so that a can be expressed in terms of its
B
tangential and normal components. At the other end of rod BC point C
moves along a straight-lined path, which is defined by the piston.
Hence, a is horizontal, Fig. 2-31,b.
C

a b
Fig. 2-32.
148