Page 153 - 4624

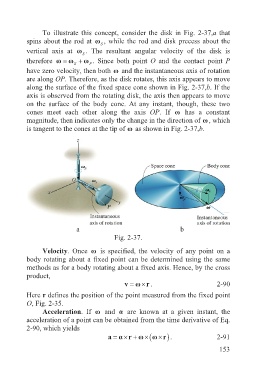
To illustrate this concept, consider the disk in Fig. 2-37,a that
spins about the rod at Ȧ , while the rod and disk precess about the
S
vertical axis at Ȧ . The resultant angular velocity of the disk is
S
therefore ȦȦ S Ȧ . Since both point O and the contact point P

P
have zero velocity, then both Ȧ and the instantaneous axis of rotation
are along OP. Therefore, as the disk rotates, this axis appears to move
along the surface of the fixed space cone shown in Fig. 2-37,b. If the
axis is observed from the rotating disk, the axis then appears to move
on the surface of the body cone. At any instant, though, these two
cones meet each other along the axis OP. If Ȧ has a constant
magnitude, then indicates only the change in the direction of Ȧ, which
is tangent to the cones at the tip of Ȧ as shown in Fig. 2-37,b.

a b
Fig. 2-37.
Velocity. Once Ȧ is specified, the velocity of any point on a
body rotating about a fixed point can be determined using the same
methods as for a body rotating about a fixed axis. Hence, by the cross
product,
v Ȧ r. 2-90
u
Here r defines the position of the point measured from the fixed point
O, Fig. 2-35.
Acceleration. If Ȧ and Į are known at a given instant, the
acceleration of a point can be obtained from the time derivative of Eq.
2-90, which yields
a u Į u Ȧ r Ȧ ur . 2-91
153