Page 147 - 4624

component of B with respect to and direction is always from B
towards A.
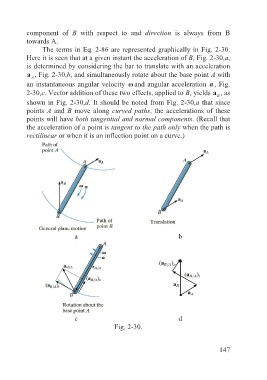
The terms in Eq. 2-86 are represented graphically in Fig. 2-30.
Here it is seen that at a given instant the acceleration of B, Fig. 2-30,a,
is determined by considering the bar to translate with an acceleration
a , Fig. 2-30,b, and simultaneously rotate about the base point A with
A
an instantaneous angular velocity ωand angular acceleration α, Fig.
2-30,c. Vector addition of these two effects, applied to B, yields a , as
B
shown in Fig. 2-30,d. It should be noted from Fig. 2-30,a that since
points A and B move along curved paths, the accelerations of these
points will have both tangential and normal components. (Recall that
the acceleration of a point is tangent to the path only when the path is
rectilinear or when it is an inflection point on a curve.)

a b

c d
Fig. 2-30.

147