Page 11 - 4498

collision the component of velocity parallel to the wall is unchanged and
the component perpendicular to the wall is reversed.
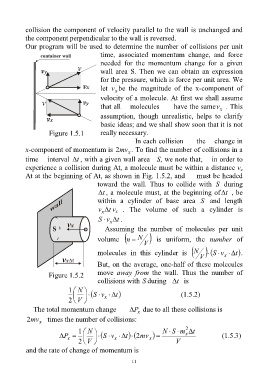
Our program will be used to determine the number of collisions per unit
time, associated momentum change, and force

needed for the momentum change for a given
wall area S. Then we can obtain an expression
for the pressure, which is force per unit area. We

let v be the magnitude of the x-component of
x
velocity of a molecule. At first we shall assume

that all molecules have the samev . This
x
assumption, though unrealistic, helps to clarify

basic ideas; and we shall show soon that it is not
Figure 1.5.1 really necessary.
In each collision the change in

x-component of momentum is mv2 x . To find the number of collisions in a
time interval t , with a given wall area S, we note that, in order to

experience a collision during At, a molecule must be within a distance v
x
At at the beginning of At, as shown in Fig. 1.5.2, and must be headed
toward the wall. Thus to collide with S during
t  , a molecule must, at the beginning of t , be

within a cylinder of base area S and length
v x t  v . The volume of such a cylinder is
x
S v  x t  .

Assuming the number of molecules per unit

volume n  N  is uniform, the number of
V
N
molecules in this cylinder is   S  v   t  .
V x
But, on the average, one-half of these molecules

move away from the wall. Thus the number of
Figure 1.5.2
collisions with S during t is

1  N 
   S  v   t  (1.5.2)
x
2  V 
The total momentum change P due to all these collisions is
x
2 mv times the number of collisions:
x

2
1  N  N  S  m x t 
P  x    S  v  t    mv 2 x   (1.5.3)
x
2  V  V
and the rate of change of momentum is

11