Page 7 - 4498

k
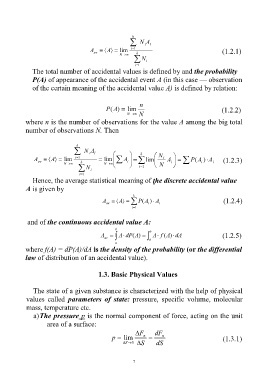
 N i A I

A av    A  lim  i 1 k (1.2.1)
N  
 N i
 i 1
The total number of accidental values is defined by and the probability
P(A) of appearance of the accidental event A (in this case — observation

of the certain meaning of the accidental value A) is defined by relation:

n
P( A  lim) (1.2.2)
N   N
where n is the number of observations for the value A among the big total
number of observations N. Then

k
 N i A I   k

A av    A  lim  i 1  lim   A i = lim  N i A i    P( A ) A i (1.2.3)




i
N   k N    N 
 N i    i 1
 i 1
Hence, the average statistical meaning of the discrete accidental value
A is given by
k
A av    A   P( A )  A (1.2.4)
i
i
 i 1

and of the continuous accidental value A:
b
b
A av  A  dP( A)   A  f ( A)  dA (1.2.5)

a a
where f(A) = dP(A)/dA is the density of the probability (or the differential

law of distribution of an accidental value).

1.3. Basic Physical Values

The state of a given substance is characterized with the help of physical
values called parameters of state: pressure, specific volume, molecular
mass, temperature etc.

a) The pressure p is the normal component of force, acting on the unit
area of a surface:

 F n dF n
p  lim  (1.3.1)
 S0  S dS

7