Page 43 - Microsoft Word - 4236

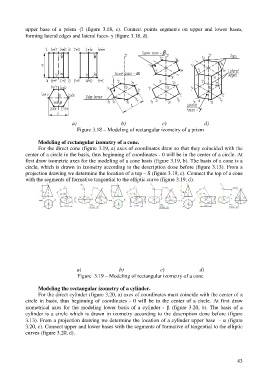
upper base of a prism - (figure 3.18, c). Connect points segments on upper and lower bases,
forming lateral edges and lateral faces-  (figure 3.18, d).

а) b) c) d)
Figure 3.18 – Modeling of rectangular іzometry of a prism

Modeling of rectangular іzometry of a cone.
For the direct cone (figure 3.19, a) axes of coordinates draw so that they coincided with the
center of a circle in the basis, thus beginning of coordinates - 0 will be in the center of a circle. At
first draw isometric axes for the modeling of a cone basis (figure 3.19, b). The basis of a cone is a
circle, which is drawn in іzometry according to the description done before (figure 3.13). From a
projection drawing we determine the location of a top – S (figure 3.19, c). Connect the top of a cone
with the segments of formative tangential to the elliptic curve (figure 3.19, d).

а) b) c) d)
Figure 3.19 – Modeling of rectangular іzometry of a cone

Modeling the rectangular іzometry of a cylinder.
For the direct cylinder (figure 3.20, a) axes of coordinates must coincide with the center of a
circle in basis, thus beginning of coordinates - 0 will be in the center of a circle. At first draw
isometrical axes for the modeling lower basis of a cylinder -  (figure 3.20, b). The basis of a
cylinder is a circle which is drawn in іzometry according to the description done before (figure
3.13). From a projection drawing we determine the location of a cylinder upper base -  (figure
3.20, c). Connect upper and lower bases with the segments of formative of tangential to the elliptic
curves (figure 3.20, d).

43