Page 42 - Microsoft Word - 4236

а) b) c)
Figure 3.16 – The modeling of a rectangular dimetry circle

Then we draw the minor axis of an ellipse (segment [CD] = 0,95  D (dash-dotted line) or
[EF] = 0,35  D) placed parallell to the axonometrical axis which doesn’t exist in this plane, and
also major axis (segment [AB] = 1,06  D) which will be perpendicular to it (figure 3.16, b). The
ellipse is traced on the eight points obtained by curve (figure 3.16, c).

3.3.2 EXAMPLES OF MODELING THE ISOMETRICAL PROJECTIONS OF
SIMPLE FIGURES

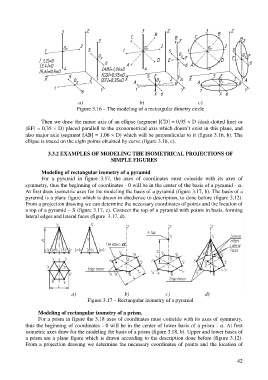
Modeling of rectangular izometry of a pyramid
For a pyramid in figure 3.17, the axes of coordinates must coincide with its axes of
symmetry, thus the beginning of coordinates - 0 will be in the center of the basis of a pyramid - .
At first draw isometric axes for the modeling the basis of a pyramid (figure 3.17, b). The basis of a
pyramid is a plane figure which is drawn in obedience to description, to done before (figure 3.12).
From a projection drawing we can determine the necessary coordinates of points and the location of
a top of a pyramid – S (figure 3.17, c). Connect the top of a pyramid with points in basis, forming
lateral edges and lateral faces (figure 3.17, d).

а) b) c) d)
Figure 3.17 – Rectangular izometry of a pyramid

Modeling of rectangular іzometry of a prism.
For a prism in figure the 3.18 axes of coordinates must coincide with its axes of symmetry,
thus the beginning of coordinates - 0 will be in the center of lower basis of a prism - . At first
isometric axes draw for the modeling the basis of a prism (figure 3.18, b). Upper and lower bases of
a prism are a plane figure which is drawn according to the description done before (figure 3.12).
From a projection drawing we determine the necessary coordinates of points and the location of

42