Page 51 - 4234
P. 51
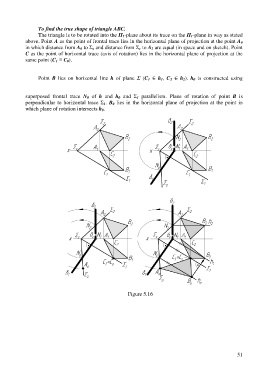
To find the true shape of triangle ABC.
The triangle is to be rotated into the Π 1-plane about its trace on the Π 1-plane in way as stated
above. Point A as the point of frontal trace lies in the horizontal plane of projection at the point A 0
in which distance from A 0 to Σ x and distance from Σ x to A 2 are equal (in space and on sketch). Point
C as the point of horizontal trace (axis of rotation) lies in the horizontal plane of projection at the
same point (C 1 ≡ C 0).
Point B lies on horizontal line h of plane Σ (C 1 ∈ h 1, C 2 ∈ h 2). h 0 is constructed using
superposed frontal trace N 0 of h and h 0 and Σ 1 parallelism. Plane of rotation of point B is
perpendicular to horizontal trace Σ 1. B 0 lies in the horizontal plane of projection at the point in
which plane of rotation intersects h 0.
Figure 5.16
51