Page 46 - 4234

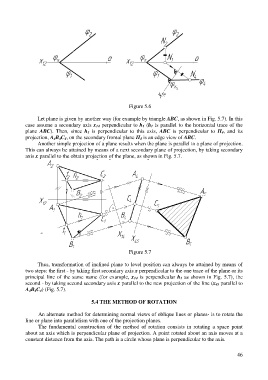
Figure 5.6

Let plane is given by another way (for example by triangle ABC, as shown in Fig. 5.7). In this
case assume a secondary axis x 14 perpendicular to h 1 (h 1 is parallel to the horizontal trace of the
plane ABC). Then, since h 1 is perpendicular to this axis, ABC is perpendicular to Π 4, and its
projection, A 4B 4C 4, on the secondary frontal plane Π 4 is an edge view of ABC.
Another simple projection of a plane results when the plane is parallel to a plane of projection.
This can always be attained by means of a next secondary plane of projection, by taking secondary
axis x parallel to the obtain projection of the plane, as shown in Fig. 5.7.

Figure 5.7

Thus, transformation of inclined plane to level position can always be attained by means of
two steps: the first - by taking first secondary axis x perpendicular to the one trace of the plane or its
principal line of the same name (for example, x 14 is perpendicular h 1 as shown in Fig. 5.7), the
second - by taking second secondary axis x parallel to the new projection of the line (x 45 parallel to
A 4B 4C 4) (Fig. 5.7).

5.4 THE METHOD OF ROTATION

An alternate method for determining normal views of oblique lines or planes- is to rotate the
line or plane into parallelism with one of the projection planes.
The fundamental construction of the method of rotation consists in rotating a space point
about an axis which is perpendicular plane of projection. A point rotated about an axis moves at a
constant distance from the axis. The path is a circle whose plane is perpendicular to the axis.

46