Page 55 - 4234

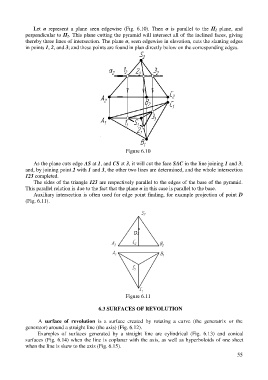
Let α represent a plane seen edgewise (Fig. 6.10). Then α is parallel to the Π 1 plane, and
perpendicular to Π 2. This plane cutting the pyramid will intersect all of the inclined faces, giving
thereby three lines of intersection. The plane α, seen edgewise in elevation, cuts the slanting edges
in points 1, 2, and 3; and these points are found in plan directly below on the corresponding edges.

Figure 6.10

As the plane cuts edge AS at 1, and CS at 3, it will cut the face SAC in the line joining 1 and 3;
and, by joining point 2 with 1 and 3, the other two lines are determined, and the whole intersection
123 completed.
The sides of the triangle 123 are respectively parallel to the edges of the base of the pyramid.
This parallel relation is due to the fact that the plane α in this case is parallel to the base.
Auxiliary intersection is often used for edge point finding, for example projection of point D
(Fig. 6.11).

Figure 6.11

6.3 SURFACES OF REVOLUTION

A surface of revolution is a surface created by rotating a curve (the generatrix or the
generator) around a straight line (the axis) (Fig. 6.12).
Examples of surfaces generated by a straight line are cylindrical (Fig. 6.13) and conical
surfaces (Fig. 6.14) when the line is coplanar with the axis, as well as hyperboloids of one sheet
when the line is skew to the axis (Fig. 6.15).
55