Page 47 - 4234

The rotation of a point about a vertical (horizontal-projecting) axis
As an illustration of the general case of this problem, let consider the rotation of a point about
a vertical (horizontal-projecting) axis ( Fig. 5.8).

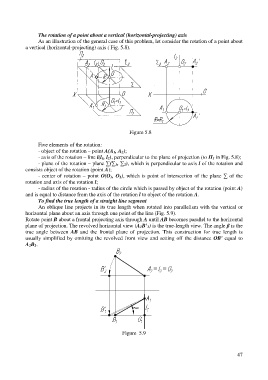
Figure 5.8

Five elements of the rotation:
- object of the rotation – point A(A 1, A 2);
- axis of the rotation – line l(l 1, l 2), perpendicular to the plane of projection (to Π 1 in Fig. 5.8);
- plane of the rotation – plane ∑(∑ 1, ∑ 2), which is perpendicular to axis l of the rotation and
consists object of the rotation (point A);
- center of rotation – point O(O 1, O 2), which is point of intersection of the plane ∑ of the
rotation and axis of the rotation l;
- radius of the rotation - radius of the circle which is passed by object of the rotation (point A)
and is equal to distance from the axis of the rotation l to object of the rotation A.
To find the true length of a straight line segment
An oblique line projects in its true length when rotated into parallelism with the vertical or
horizontal plane about an axis through one point of the line (Fig. 5.9).
Rotate point B about a frontal projecting axis through A until AB becomes parallel to the horizontal
plane of projection. The revolved horizontal view (A 1B’ 1) is the true-length view. The angle β is the
true angle between AB and the frontal plane of projection. This construction for true length is
usually simplified by omitting the revolved front view and setting off the distance OB' equal to
A 2B 2.

Figure 5.9

47