Page 52 - 4234

6 SURFACES AND SOLIDS
6.1 POLYHEDRONS

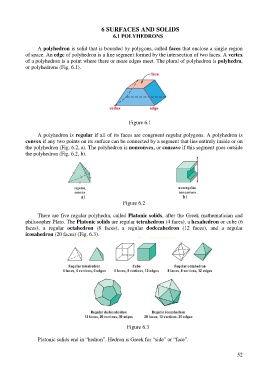
A polyhedron is solid that is bounded by polygons, called faces that enclose a single region
of space. An edge of polyhedron is a line segment formed by the intersection of two faces. A vertex
of a polyhedron is a point where there or more edges meet. The plural of polyhedron is polyhedra,
or polyhedrons (Fig. 6.1).

Figure 6.1

A polyhedron is regular if all of its faces are congruent regular polygons. A polyhedron is
convex if any two points on its surface can be connected by a segment that lies entirely inside or on
the polyhedron (Fig. 6.2, a). The polyhedron is nonconvex, or concave if this segment goes outside
the polyhedron (Fig. 6.2, b).

a) b)
Figure 6.2

There are five regular polyhedra, called Platonic solids, after the Greek mathematician and
philosopher Plato. The Platonic solids are regular tetrahedron (4 faces), a hexahedron or cube (6
faces), a regular octahedron (8 faces), a regular dodecahedron (12 faces), and a regular
icosahedron (20 faces) (Fig. 6.3).

Figure 6.3

Platonic solids end in “hedron”. Hedron is Greek for “side” or “face”.

52