Page 49 - 4234

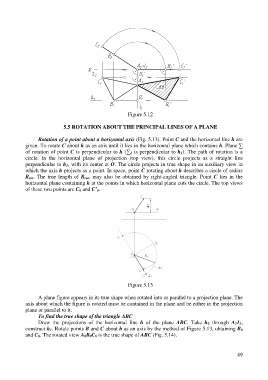
Figure 5.12

5.5 ROTATION ABOUT THE PRINCIPAL LINES OF A PLANE

Rotation of a point about a horizontal axis (Fig. 5.13). Point C and the horizontal line h are
given. To rotate C about h as an axis until it lies in the horizontal plane which contains h. Plane ∑
of rotation of point C is perpendicular to h (∑ 1 is perpendicular to h 1). The path of rotation is a
circle. In the horizontal plane of projection (top view), this circle projects as a straight line
perpendicular to h 1, with its center at O. The circle projects in true shape in an auxiliary view in
which the axis h projects as a point. In space, point C rotating about h describes a circle of radius
R rot. The true length of R rot. may also be obtained by right-angled triangle. Point C lies in the
horizontal plane containing h at the points in which horizontal plane cuts the circle. The top views
of these two points are C 0 and C’ 0.

Figure 5.13

A plane figure appears in its true shape when rotated into or parallel to a projection plane. The
axis about which the figure is rotated must be contained in the plane and be either in the projection
plane or parallel to it.
To find the true shape of the triangle ABC
Draw the projections of the horizontal line h of the plane ABC. Take h 2 through A 21 2,

construct h 1. Rotate points B and C about h as an axis by the method of Figure 5.13, obtaining B 0
and C 0. The rotated view A 0B 0C 0 is the true shape of ABC (Fig. 5.14).

49