Page 45 - 4234

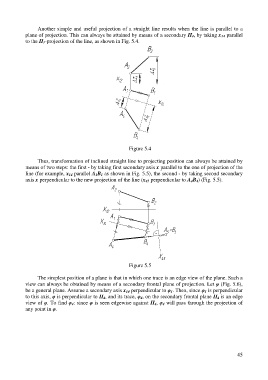
Another simple and useful projection of a straight line results when the line is parallel to a
plane of projection. This can always be attained by means of a secondary Π 4, by taking x 14 parallel
to the Π 1-projection of the line, as shown in Fig. 5.4.

Figure 5.4

Thus, transformation of inclined straight line to projecting position can always be attained by
means of two steps: the first - by taking first secondary axis x parallel to the one of projection of the
line (for example, x 14 parallel A 1B 1 as shown in Fig. 5.5), the second - by taking second secondary
axis x perpendicular to the new projection of the line (x 45 perpendicular to A 4B 4) (Fig. 5.5).

Figure 5.5

The simplest position of a plane is that in which one trace is an edge view of the plane. Such a
view can always be obtained by means of a secondary frontal plane of projection. Let φ (Fig. 5.6),
be a general plane. Assume a secondary axis x 14 perpendicular to φ 1. Then, since φ 1 is perpendicular
to this axis, φ is perpendicular to Π 4, and its trace, φ 4, on the secondary frontal plane Π 4 is an edge
view of φ. To find φ 4: since φ is seen edgewise against Π 4, φ 4 will pass through the projection of
any point in φ.

45