Page 43 - 4234

5 CONVERSION OF PROJECTION
5.1 CHANGE OF PROJECTION PLANES

The introduction of auxiliary projection planes provides another method of attack on a
problem. A general case may often be reduced to a special case having a simple solution by
choosing suitable auxiliary views. The auxiliary-view (auxiliary-projection) method of solution is
especially useful when the given data lies in an unfavorable position with respect to the projection
planes. For example, the true distance between two parallel straight lines which are inclined to the
horizontal and frontal projection plane can be measured readily in the auxiliary view in which the
lines project as points.

5.2 CHANGE OF PROJECTION PLANES (SECONDARY PLANES OF
PROJECTION)

In practical work, views of objects are often wanted in other directions than those which are
obtained by the use of the horizontal and frontal coordinate planes. In fact, it often happens that an
actual object cannot be adequately represented by a simple plan and elevation. In the theory, also,
an additional projection in a suitably chosen direction may give readily a solution otherwise
difficult to obtain. Such views, or projections, are obtained on secondary planes of projection, taken
perpendicular to either Π 1 or Π 2, and making any angle whatever with the other coordinate planes.
Secondary planes of projection are by preference taken perpendicular to Π 1, for while any
plane perpendicular to Π 1 is vertical and named secondary frontal plane of projection (Π 4, Π 6, …),
a plane perpendicular to Π 2 is neither vertical nor horizontal, and therefore does not conform to
either of the natural directions. This plane is named secondary horizontal plane of projection (Π 5,
Π 7, …).
A very important secondary plane of projection is profile plane, which is perpendicular to both
Π 1 and Π 2. This plane has just been discussed in detail in the preceding lecture. Indeed, this plane
can be considered as a third coordinate plane of equal rank with the horizontal and frontal
coordinate planes. The profile plane can be considered as a special case of a plane perpendicular to
Π 2.
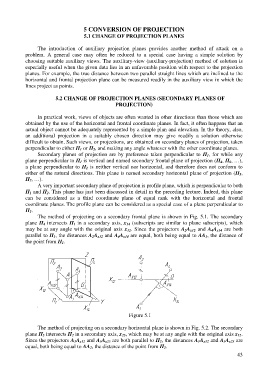
The method of projecting on a secondary frontal plane is shown in Fig. 5.1. The secondary
plane Π 4 intersects Π 1 in a secondary axis, x 14 (subscripts are similar to plane subscripts), which
may be at any angle with the original axis x 12. Since the projectors A 2A x12 and A 4A x14 are both
parallel to Π 1, the distances A 2A x12 and A 4A x14 are equal, both being equal to AA 1, the distance of
the point from Π 1.

Figure 5.1

The method of projecting on a secondary horizontal plane is shown in Fig. 5.2. The secondary
plane Π 5 intersects Π 2 in a secondary axis, x 25, which may be at any angle with the original axis x 12.
Since the projectors A 1A x12 and A 5A x25 are both parallel to Π 2, the distances A 1A x12 and A 5A x25 are
equal, both being equal to AA 2, the distance of the point from Π 2.
43